【ベストコレクション】 cos inverse 1-x^2/1 x^2 formula 137008-Cos inverse 1-x^2/1+x^2 formula

Cos (x) = −1 cos ( x) = 1 Take the inverse cosine of both sides of the equation to extract x x from inside the cosine x = arccos(−1) x = arccos ( 1) The exact value of arccos(−1) arccos ( 1) is π π x = π x = π The cosine function is negative in the second and third quadrants To find the second solution, subtract the©05 BE Shapiro Page 3 This document may not be reproduced, posted or published without permission The copyright holder makes no representation about the accuracy, correctness, orSolution Comparing this problem with the formulas stated in the rule on integration formulas resulting in inverse trigonometric functions, the integrand looks similar to the formula for tan −1 u C tan −1 u C So we use substitution, letting u = 2 x, u = 2 x, then d u = 2 d x d u = 2 d x and

1

Cos inverse 1-x^2/1+x^2 formula

Cos inverse 1-x^2/1+x^2 formula-Click here👆to get an answer to your question ️ The equation 2cos ^1x sin ^1x = 11pi6 hasSolution Divide both sides of the equation by 3 arcsin (x) = (π / 2) / 3 arcsin (x) = π / 6 Apply sin to both sides and simplify sin (arcsin (x)) = sin (π / 6) The above simplify to x = 1 / 2 Because of the domain of arcsin (x), we need to verify that the solution obtained is valid

2 Tan 1x ただの悪魔の画像

Cos (x)= 1/2 \square!According to this formula, the expression in the numerator can be simplified d d x ( cos − 1 ⁡ x) = lim h → 0 sin − 1 ⁡ ( x 1 − ( x h) 2 − ( x h) 1 − x 2) h The limit of the inverse trigonometric function gives us the indeterminate form when we try to evaluate the function by direct substitution as h approaches zeroCos = b c;

Cos(ˇ ) = cos 6 tgHere is an example Example 1 Evaluate cos 1 (1/2) If y = cos 1 (1/2), then cos y = 1/2 This equation has an infinite number of solutions, but only one of them is in the range of cos 1 x1 Inform you about time table of exam 2 Inform you about new question papers 3 New video tutorials information

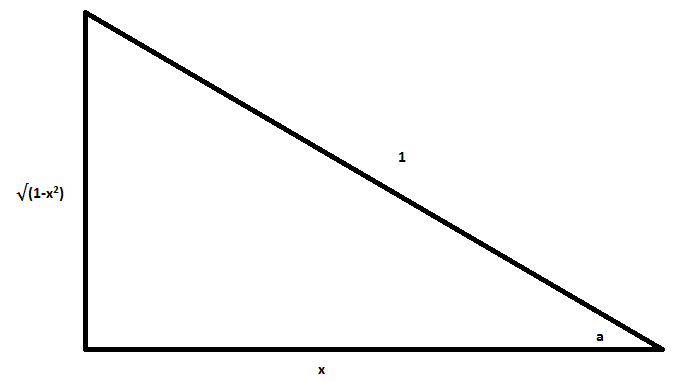
As claimed 2221 Example Find the derivative of each of the following functions Ex 53, 11 Find 𝑑𝑦/𝑑𝑥 in, 𝑦 = cos–1 ((1− 𝑥^2)/( 1 𝑥2 )) , 0 < x < 1 𝑦 = cos–1 ((1− 𝑥^2)/( 1 𝑥2 )) Putting x = tan θ ySince the domain of sin 1 x is 1 to 1 for the values of x Example 2 Find the value of sin1(sin (π/6))

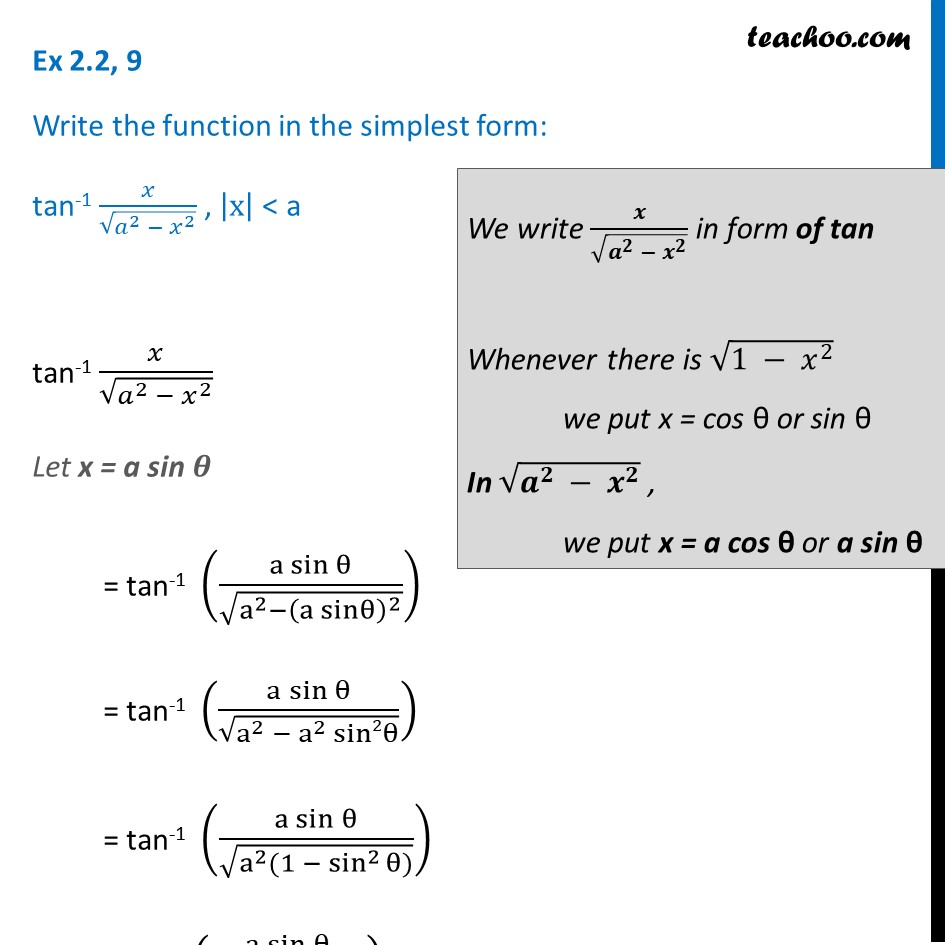
Ex 2 2 9 Class 12 Inverse Cbse Tan 1 X Root X2

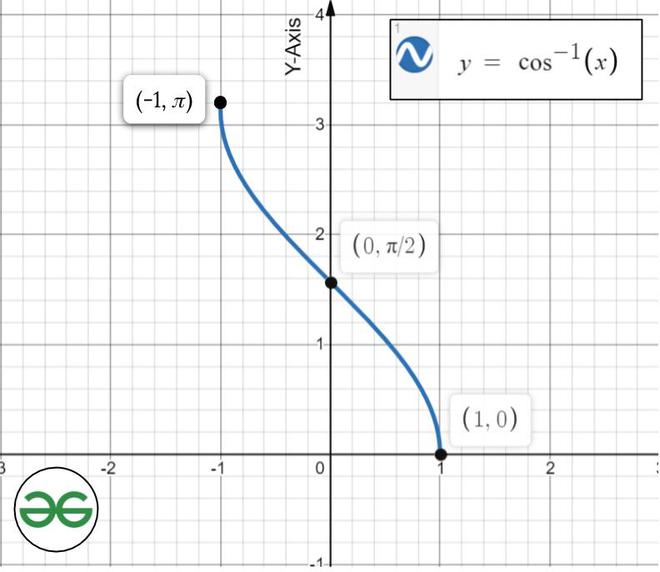
Graphs Of Inverse Trigonometric Functions Trigonometry Class 12 Maths Geeksforgeeks

Why create a profile on Shaalaacom?Tg = a b;Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more

Q Solve Cos Inverse X 2 1 X 2 1 Tan Inverse 2x X 2 1 2pi 3 Maths Inverse Trigonometric Functions Meritnation Com

Chapter 4 Maths 3

Free functions inverse calculator find functions inverse stepbystep This website uses cookies to ensure you get the best experience By using this website, you agree to our Cookie Policy Evaluating y = cos 1 x Evaluating cos 1 x expressions follows the same procedure as evaluating sin 1 x expressionsyou must be aware of the domain and range of the function!Answer (1 of 6) > What is cos (tan^1 x) =?

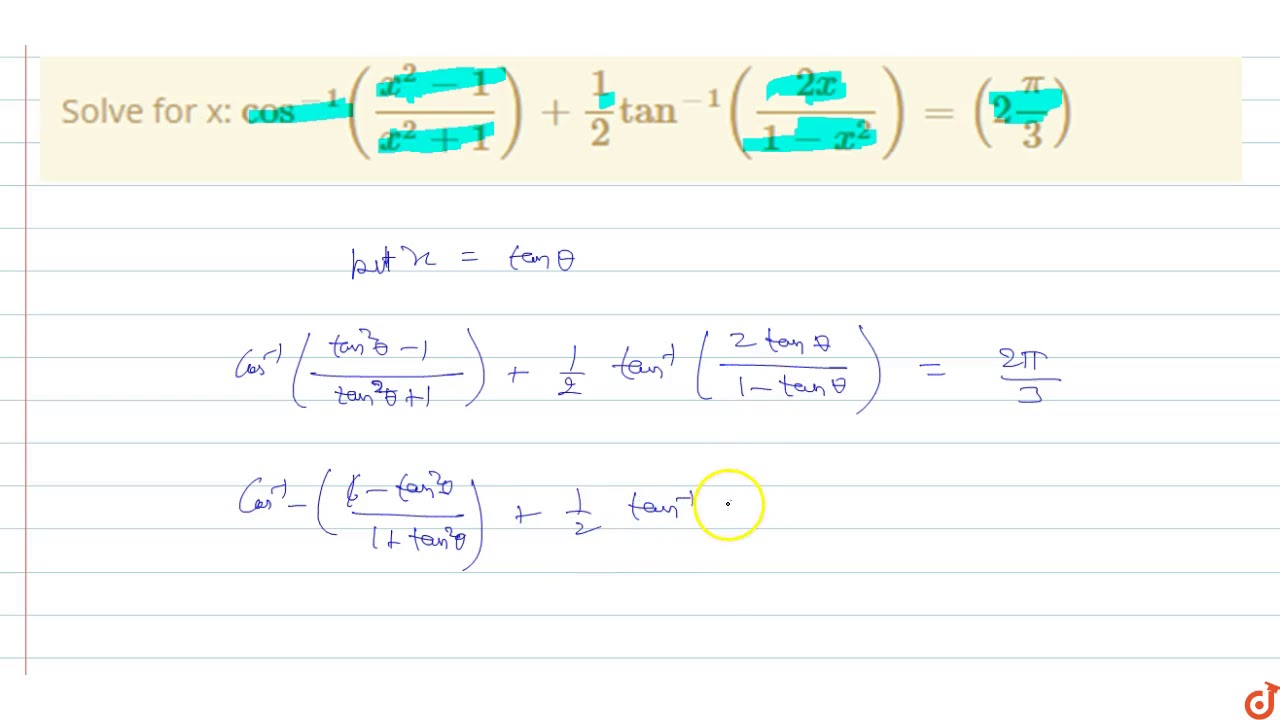
Solve For X Cos 1 X 2 1 X 2 1 1 2tan 1 2x 1 X 2 2pi 3 Youtube

Find The Greatest And Least Values Of Sin Inverse X Square Cos Inverse X Square Maths Inverse Trigonometric Functions Meritnation Com

The differentiation of the cos inverse function can be written in any variable Here are few examples to learn how to write the formula for the derivative of cosine inverse function in differential calculus ( 1) d d z ( cos − 1 ⁡ ( z)) = − 1 1 − z 2 ( 2) d d u ( cos − 1 ⁡ ( u)) = − 1 1 − u 2 ( 3) d d y ( cos − 1Click here👆to get an answer to your question ️ The equation 2 cos^1x = cos^1(2x^2 1) is satisfied by Join / Login >> Class 12 >> Maths >> Inverse Trigonometric Functions >> Identities Related to Inverse Trigonometric Functions >> The equation 2 cos^1x = co Question The equation 2 cos − 1 x = cos tan1 x = y x= tany 2tan1 x= cos1 1x 2 /1x 2 = cos1 1tan 2 y/1tan 2 y = cos1 (cos2y) =2y =2tan1 x therefore, 2tan1 x = cos1 1x 2 /1x 2

1

Cos 1 1 2x 2 Sqrt 1 X 2 Sqrt 1 X 2 4 Cos 1 X 2 Cos 1 X

X 2 x 1 − x 2 = − 2 From LMVT, this is not possible , as the LHS must be − sin ⁡ x for some point x, which must have absolute value less than 1 Hence, f ( x 1) = f ( x 2) x 1 = x 2 Hence, function is invertible Now that f − 1 ( x) exists, we calculate it for x = 1 This is equivalent to solving the equation 2 x cosList of trigonometric identities In mathematics, trigonometric identities are equalities that involve trigonometric functions and are true for every value of the occurring variables for which both sides of the equality are defined Geometrically, these are identities involving certain functions of Solve for x cos^1((x^2 1)/(x^2 1)) tan^1(2x/(x^2 1) = 2π/3 asked in Mathematics by Radhika01 ( 631k points) inverse trigonometric functions

How Do You Simplify Sin Cos 1 X Socratic

Define Cos 1 1 X 2 1 X 2 In Terms Of Tan 1 X

MATH 1A HOW TO SIMPLIFY INVERSE TRIG FORMULAS PEYAM RYAN TABRIZIAN Sample Problem (1665) Show cos(sin 1(x)) = p 1 x2 1 HOW TO WRITE OUT YOUR ANSWER = 1 x2 cos(sin 1(x)) = p 1 x2 Now the question is Which do we choose, p 1 x2, or p 1 Solve, for x cos1 x sin1 (x/2) = π/6 Welcome to Sarthaks eConnect A unique platform where students can interact with teachers/experts/students to get solutions toCos(x)=1/2 Take the inverse cosine of both sides of the equation to extract from inside the cosine The exact value of is The cosine function is positive in the first and fourth quadrants To find the second solution,

1

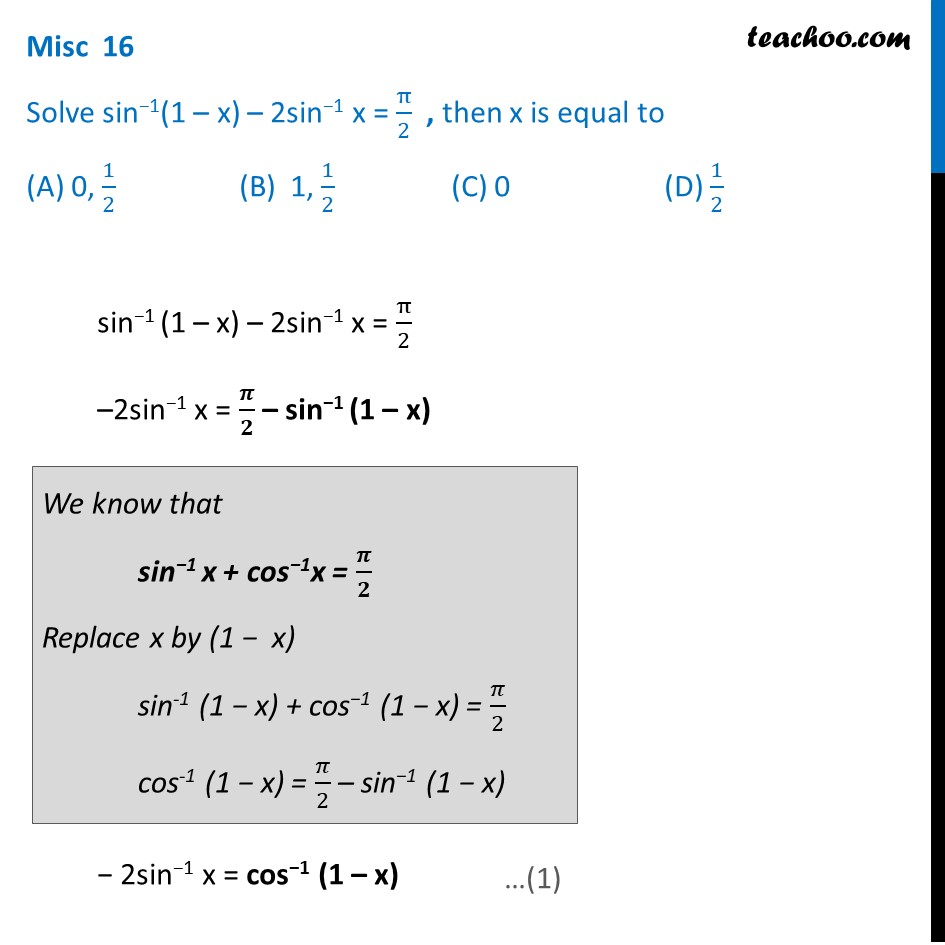
Solve Sin 1 1 X 2 Sin 1 X Pi 2 Then X Is Trigonometry Mcq

Inverse Trigonometric Functions Problems Example 1 Find the value of x, for sin (x) = 2 x =sin 1 (2), which is not possible Hence, there is no value of x for which sin x = 2;We can go directly to the formula for the antiderivative in the rule on integration formulas resulting in inverse trigonometric functions, and then evaluate the definite integral We have ∫1 0 dx √1−x2 =sin−1x1 0 =sin−11−sin−10 = π 2 −0 = π 2 ∫ 0 1 d x 1 − x 2 = sin − 1 x 0 1 = sin − 1 1 − sin − 1 0 = π 2•Step 2 Solve this equation for x in terms of y (if possible) •Step 3 To express f1 as a function of x, interchange x and y More Inverse Functions •Inverse Cosine function cos 1x=y => cos y=x and 0

Class 12 Maths Ncert Solutions Chapter 2 Inverse Trigonometric Functions Exercise 2 2

Solved 103 104 Identities Involving Inverse Trigonometric Functions Prove The Identity 2 Tan 1 Left Frac 1 X Right Cos 1 Left Frac X 2 1 X 2 1 Right Hint Let U Tan 1 Left Frac 1 X Right So That X Frac 1 Tan U

X y x y y x b)cos cos cos 1 1 1 1 1 2 2 they are valid only for some values of 'x' for which inverse trigonometric functions are well defined!22 DERIVATIVE OF INVERSE FUNCTION 3 have f0(x) = ax lna, so f0(f 1(x)) = alog a x lna= xlna Using the formula for the derivative of an inverse function, we get d dx log a x = (f 1)0(x) = 1 f0(f 1(x)) 1 xlna;Prove Sin Inverse XCos Inverse X = Pie/2Watch More Videos at https//wwwtutorialspointcom/videotutorials/indexhtmLecture By Er Ridhi Arora, Tutorials

2 Tan 1x ただの悪魔の画像

Graphs Of Inverse Trigonometric Functions Trigonometry Class 12 Maths Geeksforgeeks

Answer \;\cos\left(tan^{1}x\right)=\frac{1}{\sqrt{1x^{2}}} Let \;\theta=\tan^{1}x So, \tan\theta=x To represent this, we can draw a right triangle with the opposite side x and the adjacent side 1 Using the Pythagorean Theorem we calculate theAnswer (1 of 3) 2cot^1(x) = cot ^1(x) cot^1(x) = cot^1(xx1)/(xx) 2 cot ^1(x) = cot^1 (x^21)/(2x) AnswerSolve the Following Equation For X `3sin^1 (2x)/(1X^2)4cos^1 (1x^2)/(1X^2)2tan^1 (2x)/(1x^2)=Pi/3`

Ex 2 1 5 Find Principal Value Of Cos 1 1 2 Inverse

Solve For X Cos 1 X Sin 1 X 2 P 6 Sarthaks Econnect Largest Online Education Community

 Inverse Trigonometric Functions (Inverse Trig Functions) Inverse trig functions sin1 x , cos1 x , tan1 x etc denote angles or real numbers whose sine is x , whose cosine is x and whose tangent is x, provided that the answers given are numerically smallest available These are also written as arc sinx , arc cosx etc If there are two angles one positive & the other negativeWe shall find the integration of cosine inverse by using the integration by parts method The integration of cosine inverse is of the form I = ∫ cos – 1 x d x When using integration by parts it must have at least two functions, however this has only one function cos – 1 x So consider the second function as 1INVERSE TRIGONOMETRIC FUNCTIONS 23 Therefore, tan(cos–1x) = 1–cos θ 21– tanθ = cosθ x x = Hence 2 –1 8 1– 8 17 15 tan cos = 17 8 8 17 = Example 11 Find the value of –1 –5

Find The Range Of Sin 1 X 2 Cos 1 X 2 Mathematics Topperlearning Com 8f0ac1mm

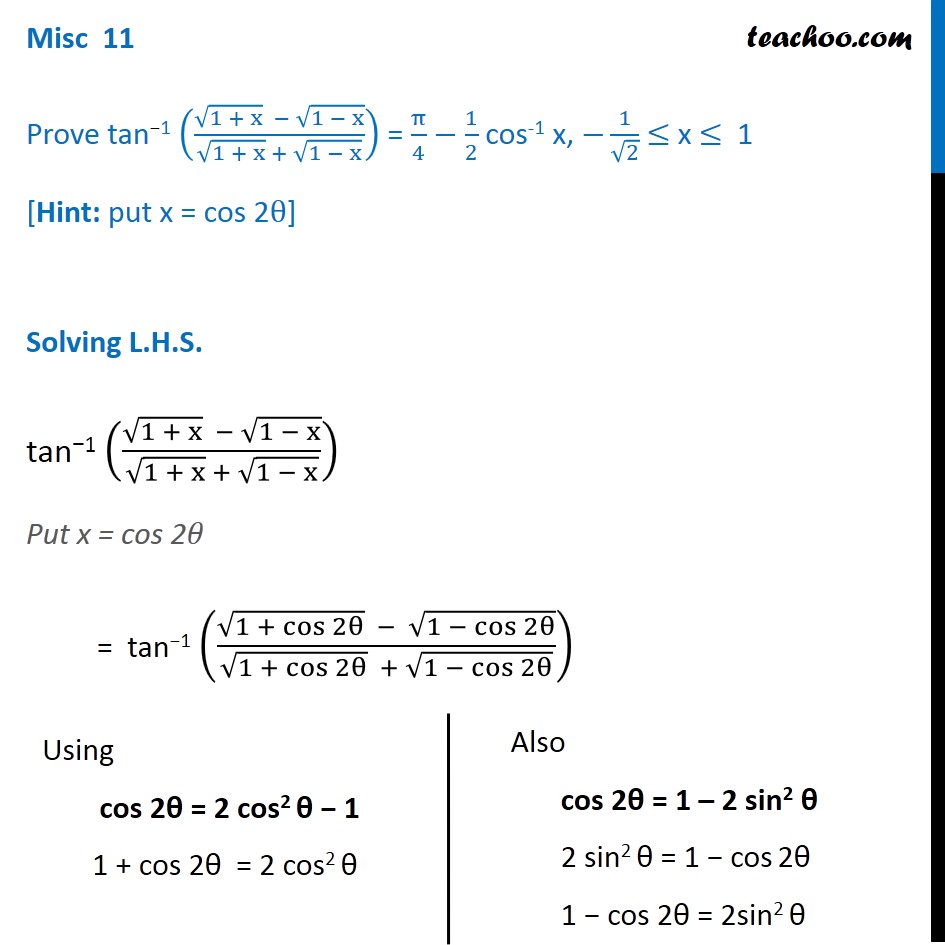
Misc 9 Prove Tan 1 Root X 1 2 Cos 1 1 X 1 X

 Ex 57, 12 If y= 〖𝑐𝑜𝑠〗^(−1) 𝑥 , Find 𝑑2𝑦/𝑑𝑥2 in terms of 𝑦 aloneLet y = 〖𝑐𝑜𝑠〗^(−1) 𝑥 Differentiating 𝑤𝑟Cos X = adjacent side/Hypotenuse Therefore, Cos 1 (Adjacent side/Hypotenuse) = X Here, the angle X is represented in degrees This is how the Cos inverse X formula is generated and used in the restricted domains Once you have understood the formula of this expression, you can easily identify the Sin Cos Tan inverse formula of any ratioCtg = cos sin 3 tg ctg = 1 4 sin ˇ 2 = cos ;

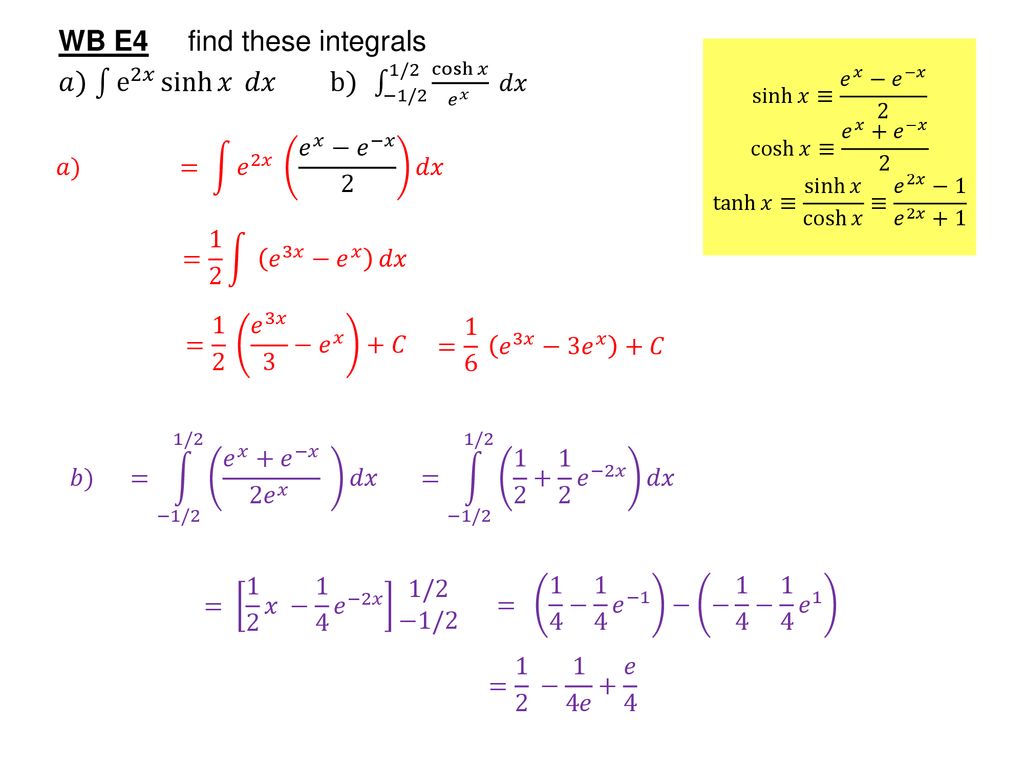
Hyperbolic Functions Ppt Download

Cos Inverse 1 X 2 1 X 2 Formula Resepahmantab

 x=sqrt80/9 To solve cos(sin^1(x))= 1/9 for x, let us assume x=sintheta and then sin^1x=theta and hence cos(sin^1(x)) = costheta=sqrt(1sin^2theta) = sqrt(1x^2) As such sqrt(1x^2)=1/9 1x^2=1/81 or x^280/81=0 or (xsqrt80/9)(xsqrt80/9)=0 Hence, x=sqrt80/9In mathematics, the inverse trigonometric functions (occasionally also called arcus functions, antitrigonometric functions or cyclometric functions) are the inverse functions of the trigonometric functions (with suitably restricted domains)Specifically, they are the inverses of the sine, cosine, tangent, cotangent, secant, and cosecant functions, and are used to obtain an angle from any ofSin(ˇ ) = sin 5 cos ˇ 2 = sin ;

Prove That Cos 1 1 2x 2 2sin 1x

Prove That Tan 1 1 X2 1 X2 1 X2 1 X2 P 4 1 2 Cos 1x2 Studyrankersonline

 Number of solution of the equation $ \cot^{1}{\sqrt{4x^2} \cos^{1}{(x^25)}}=3\pi/2$ $$ \cot^{1}{\sqrt{4x^2} \cos^{1}{(x^25)}}={3π/2}$$ Taking sine both side and solving this is I get $$1 \sqrt{5x^2}x^24 \sqrt{5x^2}\sqrt{4x^2}\sqrt{x^615x^474x^21}=0$$ After this, I can't solve it and my approach is time taking also, so plz suggest me aB catetele, c ipotenuza triunghiului dreptunghic, unghiul, opus catetei a)2 tg = sin cos ;Section 48 Derivatives of Inverse Functions Suppose we wanted to find the derivative of the inverse, but do not have an actual formula for the inverse function?Then we can use the following derivative formula for the inverse evaluated at \(a\text{}\) Theorem 480 Derivative of Inverse

Solved Derive The Following Formulas For The Inverse Chegg Com

What Is The Value Of Tan 1 2 Cos Inverse 5 3 Quora

Ctg = b a;This calculus video tutorial shows you how to find the derivatives if inverse trigonometric functions such as inverse sin^1 2x, tan^1 (x/2) cos^1 (x^2) taGet stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us!

Solve For X Cos Inverse X Square Minus One Upon X Square 1 1 2 Tan Inverse Minus 2 Maths Inverse Trigonometric Functions Meritnation Com

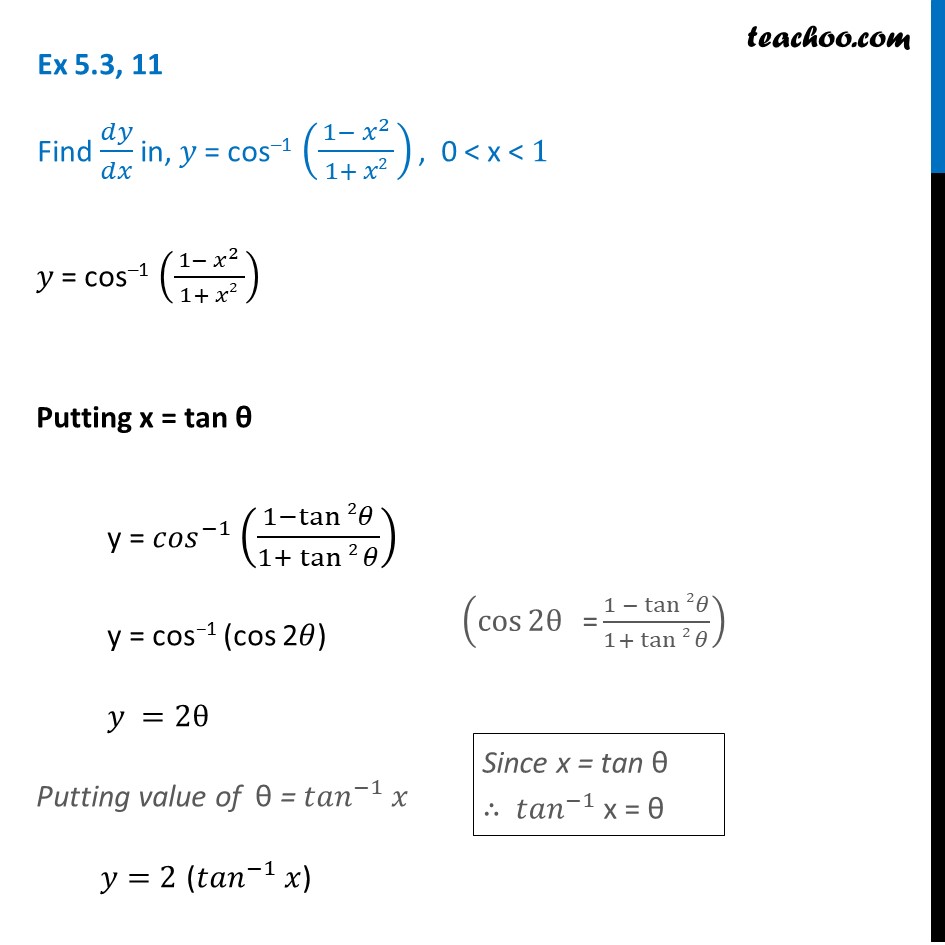
Ex 5 3 11 Find Dy Dx In Y Cos 1 1 X2 1 X2 Ex 5 3

 d/dxcos^(1)(x) = 1/sqrt(1 x^2) When tackling the derivative of inverse trig functions I prefer to rearrange and use Implicit differentiation as I always get the inverse derivatives muddled up, and this way I do not need to remember the inverse derivatives If you can remember the inverse derivatives then you can use the chain ruleCos 2α = 2x 2 1 Therefore, 2α = cos − 1 (2x 2 1) 2 cos − 1 x = cos − 1 (2x 2 1) or, 2 arccos (x) = arccos (2x 2 1) Proved Inverse Trigonometric Functions General and Principal Values of sin − 1 x General and Principal Values of cos − 1 xFormule trigonometrice 1 sin = a c;

Tan Inverse 2x 1 X Square Cot Inverse 1 X Square 2x 2pi 3 Brainly In

Ex 2 2 13 Inverse Trigonometry Tan 1 2 Sin 1 2x 1 X2

KCET 4 Let P = a i j be a 3 × 3 matrix and let Q = b i j where b i j = 2 i j a i j for 1 ≤ i, j ≤ If the determinant of P is 2, then the determinant of the matrix Q is IIT JEE 12 Determinants 5 If the sum of n terms of an AP is given by S n = n 2 n, then the common difference of the AP is Inverse Trigonometric Formulas Trigonometry is a part of geometry, where we learn about the relationships between angles and sides of a rightangled triangleIn Class 11 and 12 Maths syllabus, you will come across a list of trigonometry formulas, based on the functions and ratios such as, sin, cos and tanSimilarly, we have learned about inverse trigonometry concepts also65 Summary of Inverse Functions Here we collect the important results of this module We first recall the definitions of the inverse trigonometric functions For x in the interval 1 , 1, cos1 (x) is the angle measure in the interval 0 , whose cosine value is x

Solve For X Cos 1 X Sin 1 X 2 P 6 Sarthaks Econnect Largest Online Education Community

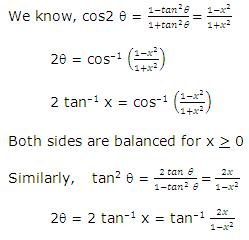
Inverse Circular Function Study Material For Iit Jee Askiitians

Prove That 1 2tan 1x Cos 1xsqrt 1 Sqrt 1 X 2 2sqrt 1 X 2 Youtube

Solved Derive The Following Formulas For The Inverse Chegg Com

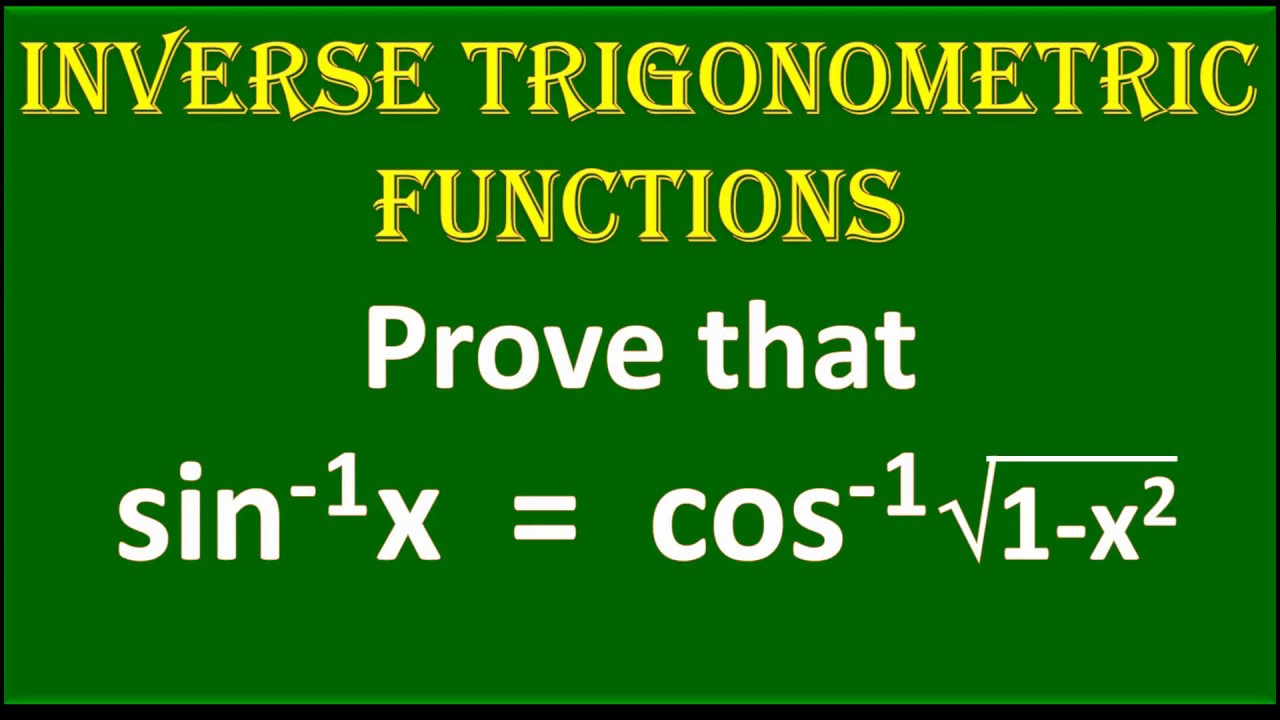
5 Prove That Sin 1x Cos 1 Root 1 X2 Inverse Trigono For Class 11 Class 12 Diploma Youtube

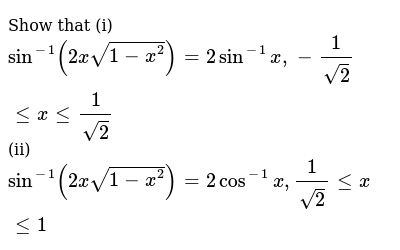
Show That I Sin 1 2xsqrt 1 X 2 2sin 1 X 1 Sqrt 2 Lt Xlt 1 Sqrt 2 Ii Sin 1 2xsqrt 1 X 2 2cos 1 X 1 Sqrt 2 Lt Xlt 1

2

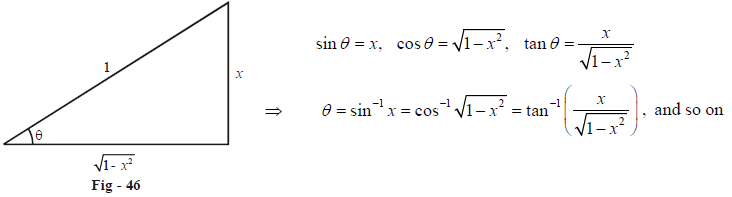
Interconversion Between Inverse Trigonometric Ratios What Is Interconversion Between Inverse Trigonometric Ratios Examples Solutions Cuemath

Prove That Cos 1 X 2 Sin 1 Sqrt 1 X 2 2 Cos 1 Sqrt 1 X 2 Youtube

How To Prove That Math Sin 1 X Cos 1 X Frac Pi 2 Math Quora

Integral Int 1 1 Frac1x Sqrt Frac 1 X 1 X Ln Left Frac 2 X 2 2 X 1 2 X 2 2 X 1 Right Mathrm Dx Mathematics Stack Exchange

The Value Of 2cos 1x Is Maths Questions

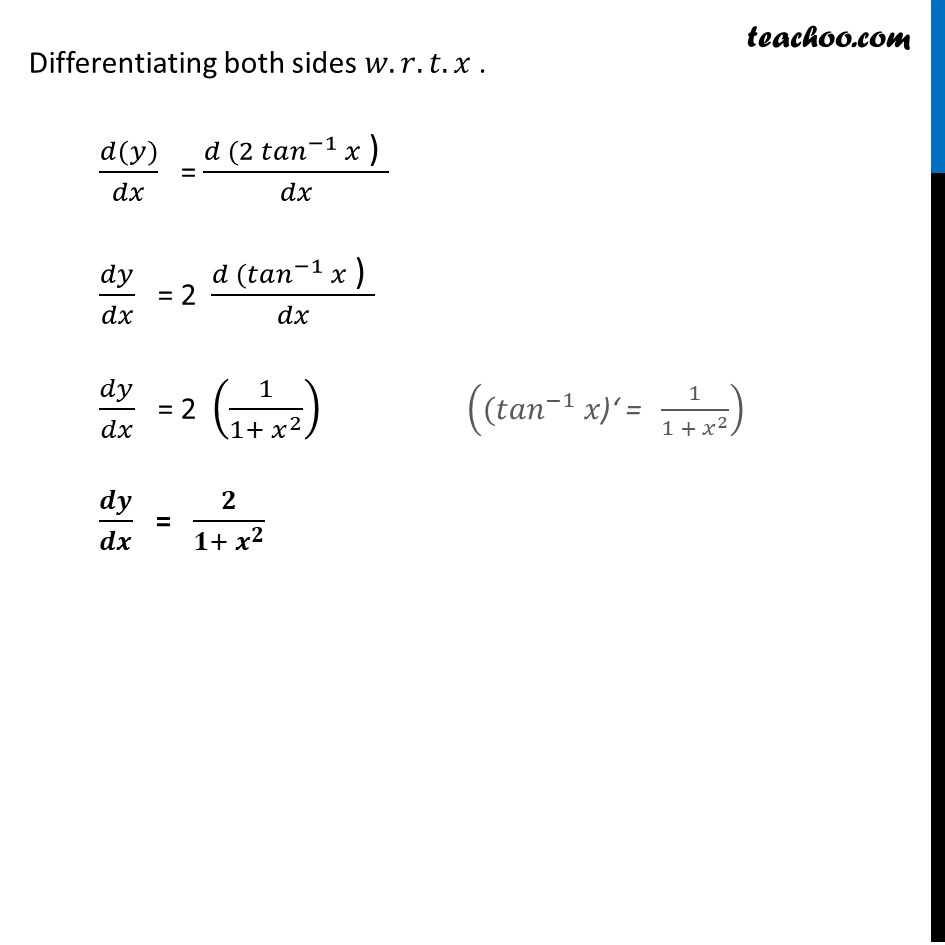
Ex 5 3 11 Find Dy Dx In Y Cos 1 1 X2 1 X2 Ex 5 3

Inverse Trigonometric Functions Precalculus Ii

Cos Inverse 1 X 2 1 X 2 Formula Resepahmantab

3

Cos Inverse 1 X 2 1 X 2 Formula Resepahmantab

Interconversion Between Inverse Trigonometric Ratios What Is Interconversion Between Inverse Trigonometric Ratios Examples Solutions Cuemath

Solve For X 2tan 1x Cos 1 1 A 2 1 A 2 Cos 1 1 B 2 1 B 2 A 0 B 0 Sarthaks Econnect Largest Online Education Community

Show That Sinh 1 X Ln X Sqrt X 2 1 Mathematics Stack Exchange

If Sin 1 2a 1 A 2 Cos 1 1 B 2 1 B 2 Tan 1 2x 1 X 2 Then Prove That X A B 1 Ab Sarthaks Econnect Largest Online Education Community

Ex 2 2 5 Simplify Tan 1 Root 1 X2 1 X Chapter 2

Differentiate Tan 1 1 X 2 X With Respect To Cos 1 2x 1 X 2 When X 0 Sarthaks Econnect Largest Online Education Community

Inverse Hyperbolic Functions Wikipedia

Proving That Cos Arcsin X Sqrt 1 X 2 Mathematics Stack Exchange

Prove That Tan 1x Cot 1 X 1 Tan 1 X 2 X 1

Simplify Cot Inverse 1 X 2 1 Brainly In

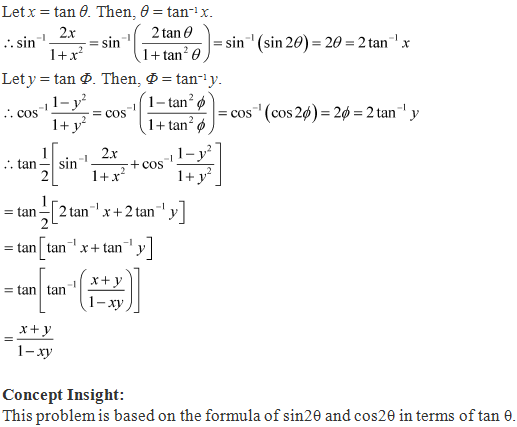
Tan1 2 Sin 1 2x 1 X 2 Cos 1 1 Y 2 1 Y 2 X Y 1 Xy If X Lt 1 Y Gt 0 And Xy Gt 1 Mathematics Topperlearning Com Exkwfcdd

Cos Inverse 1 X 2 1 X 2 Formula Resepahmantab

If Math Sqrt 1 X 2 Sqrt 1 Y 2 A X Y Math How Can I Prove Math Frac Mathrm D Y Mathrm D X Sqrt Frac 1 Y 2 1 X 2 Math Quora

2

Prove That 1 2 Tan 1 X Cos 1 1 Root 1 X2 2 Maths Inverse Trigonometric Functions Meritnation Com

Cos 2x 1 2cos X 0 Lt X Lt 1 Prove It Brainly In

Differentiate Tan 1 1 X2 1 2 1 X Wrt Sin 1 2x 1 X2 Explain In Great Detail Mathematics Topperlearning Com Yx862gdd

How Do You Evaluate Cos 1 1 2 Socratic

Solve The Following For X Cos 1 X 2 1 X 2 1 Tan 1

Integration Calculus Notes

If Cos 1 X Cos 1 Y Cos 1 Z Pie Prove That X2 Y2 Z2 2xyz 1 Sir Please Solve The Above Problem Only Maths Inverse Trigonometric Functions Meritnation Com

If Sin 1 2a 1 Cos 1 1 1 Tan 1 2x 1 X2 Where A X 0 1 Then The Value Of X Is Studyrankersonline

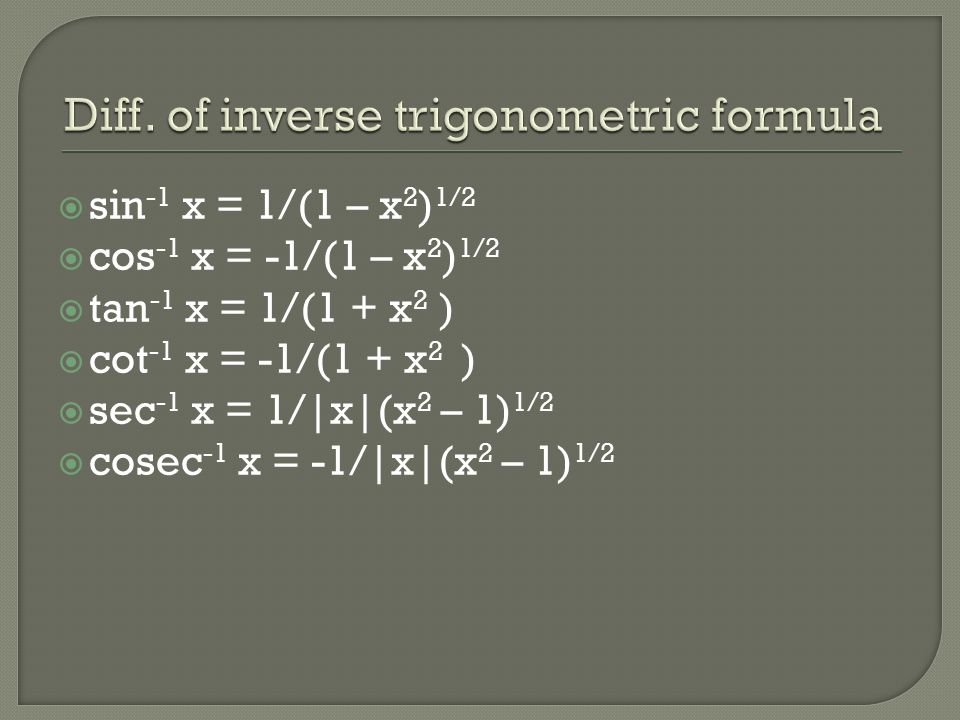
Content What Is Derivation Derivation Of Trigonometry Function Derivation S Rules Ppt Download

Ex 2 2 6 Simplify Tan 1 1 Root X2 1 Class 12 Inverse

Fhxrodp1z Bjm

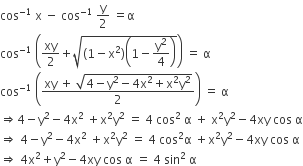
If Cos 1 X Cos 1 Y 2 A Then 4x2 4xy Cos A Y2 Is Equal To From Mathematics Inverse Trigonometric Functions Class 12 Haryana Board English Medium

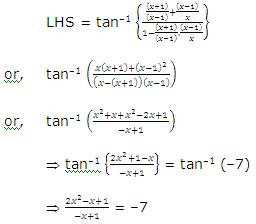
Inverse Circular Function Study Material For Iit Jee Askiitians

What Is The Integration Of Cot 1 1 X X 2 Dx Limits From 1 To 0 Quora

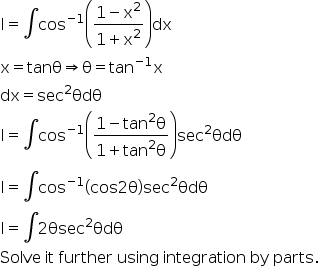
Integration Cos 1 1 X2 1 X2 Dx Explain In Great Detail Mathematics Topperlearning Com Vldkdqvv

Differentiate Tan 1 1 X2 1 2 X Wrt Cos 1 2x 1 X2 1 2 When X 0 Explain In Great Detail Mathematics Topperlearning Com Hpg5zbkk

Derivatives Of Inverse Trigonometric Functions

Cos 1x Sin 1 1 X 2 Arccos X Arcsin Sqrt 1 X2 How To Prove Formula Youtube

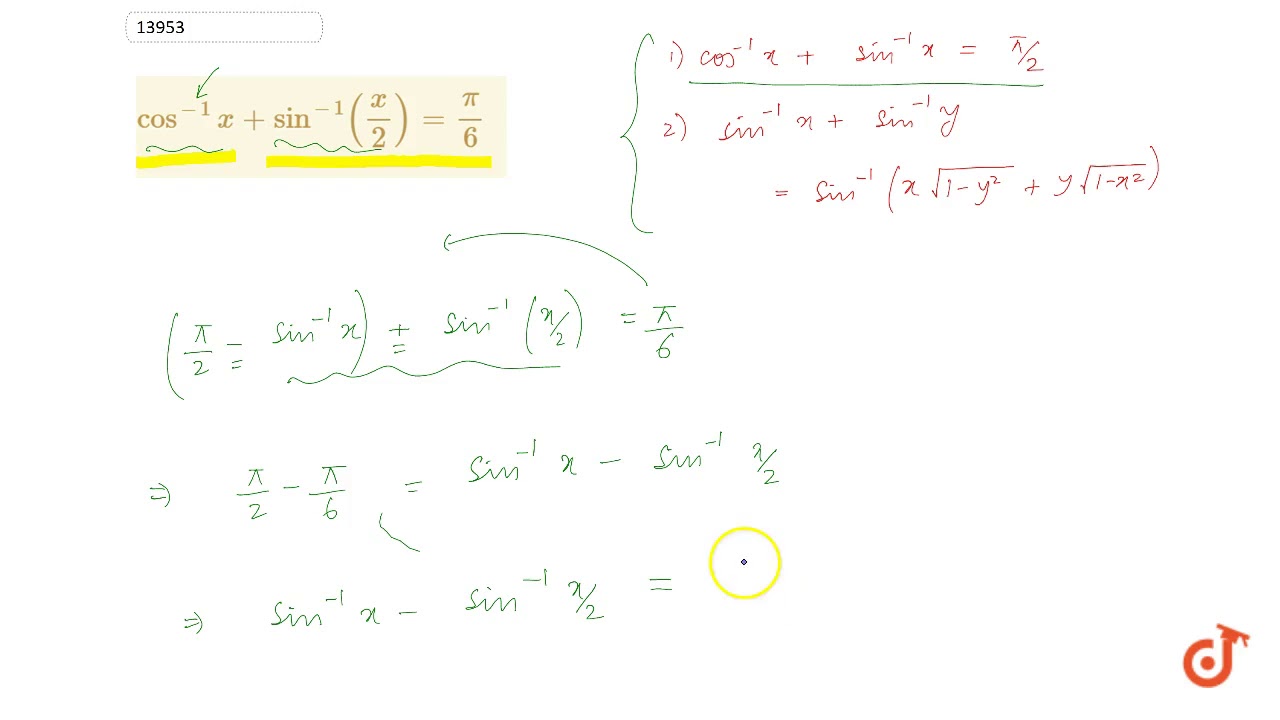
Cos 1 X Sin 1 X 2 Pi 6 Youtube

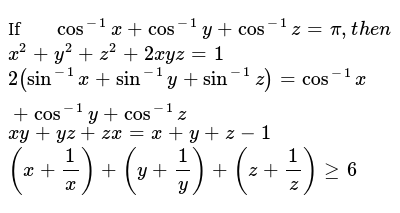
If Cos 1 X Cos 1 Y Cos 1 Z Pi T H E N X 2 Y 2 Z 2 2x Y Z 1 2 Sin 1 X Sin 1 Y Sin 1 Z Cos 1 X Cos 1 Y Cos 1 Z X Y Y Z Z X X Y Z 1 X 1 X Y 1 Y Z 1 Z Geq6

If Cos 1 X A Cos 1 Y B A Then Prove That X 2 A 2 2 Xy Ab Cos A Y 2 B 2 Sin 2 A Sarthaks Econnect Largest Online Education Community

Solved Prso Cosy Xi Any 6 Chegg Com

Derivative Of Inverse Cosine Video Khan Academy

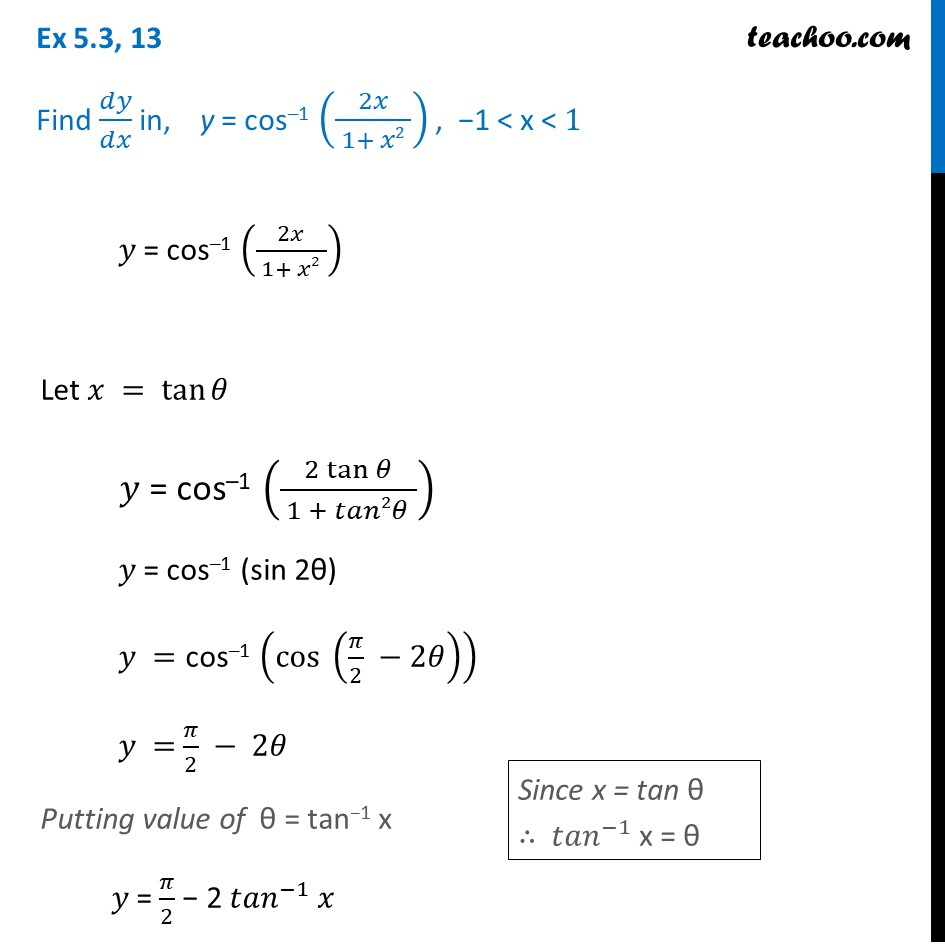
Ex 5 3 13 Find Dy Dx In Y Cos 1 2x 1 X2 Ncert Ex 5 3

Example 5 Verify A Trigonometric Identity Verify The

How To Find Derivative Of Y Sin 2x 1 X 2 Quora

Solve The Following For X Cos 1 X 2 1 X 2 1 Tan 1 2x X 2 1 2p 3 Sarthaks Econnect Largest Online Education Community

Differentiate Cos 1 1 X 2 1 X 2 With Respect To Tan 1 3x X 3 1 3x 2

Integrate Sin 1x 1 X2 3 2 Dx Studyrankersonline

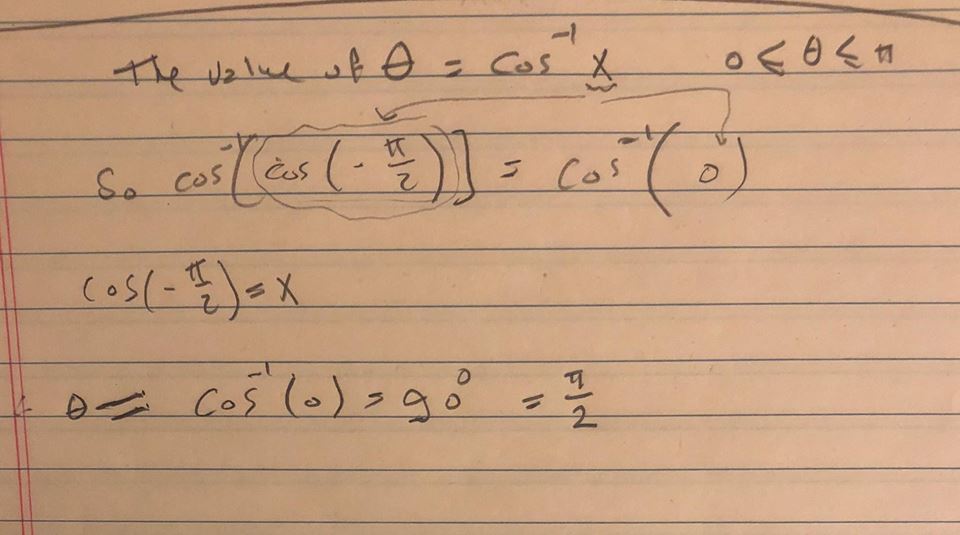
How Do You Evaluate Cos 1 Cos Pi 2 Socratic

2 Sin Inverse X Formula In Terms Of Cos Elton Reynold

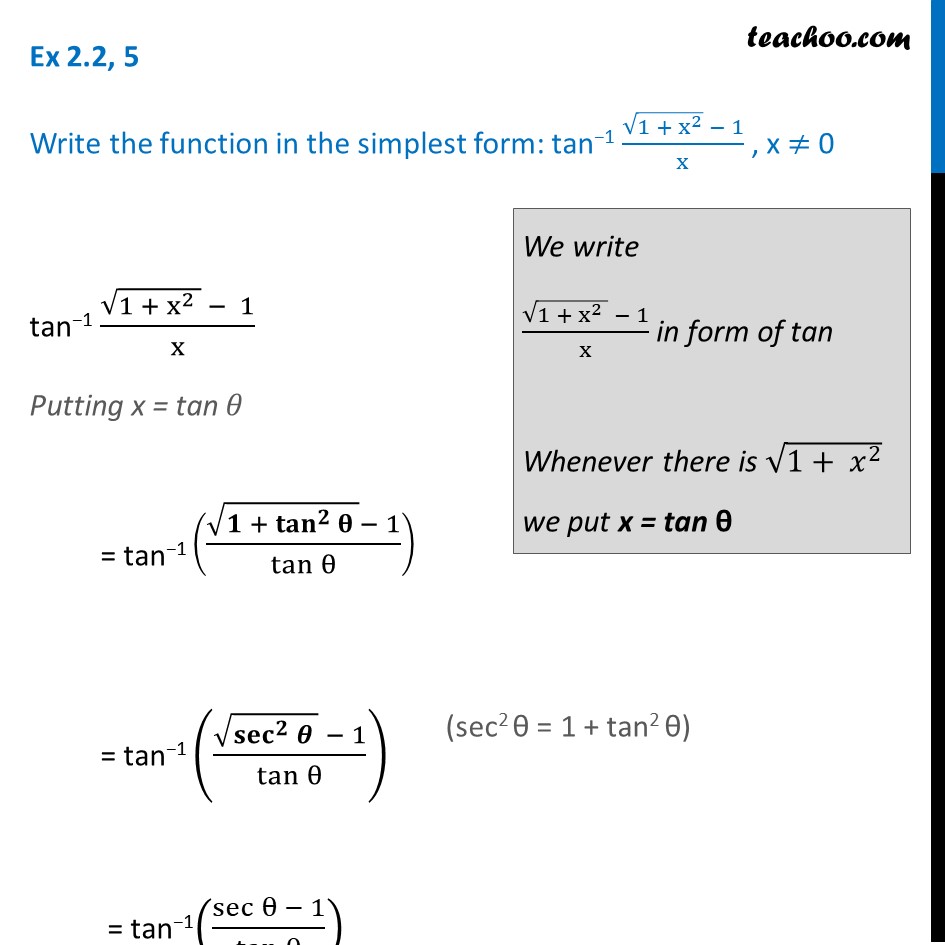
Ex 2 2 5 Simplify Tan 1 Root 1 X2 1 X Chapter 2

If Cos 1x Cos 1y Cos 1z P Prove That X2 Y2 Z2 2 X Y Z 1 From Mathematics Inverse Trigonometric Functions Class 12 Cbse

Solve For X Sin Cot 1 X 1 Cos Tan 1x Mathematics Topperlearning Com 4ntoqsll

D Dx

If Cos 1 X A Cos 1 Y B Alpha Prove That X 2 A 2 2 X Y A B Cosalpha Y 2 B 2 Sin 2al Youtube

Prove 3sin 1x Sin 1 3x 4x 3 X 1 2 1 2

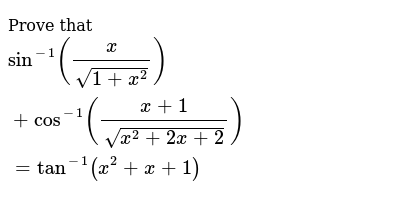
Prove That Sin 1 X Sqrt 1 X 2 Cos 1 X 1 Sqrt X 2 2x 2 Tan 1 X 2 X 1

Ex 5 7 12 If Y Cos 1 X Find D 2y Dx 2 In Terms Of Y Alone

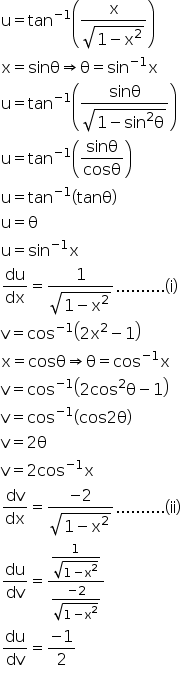
Differentiate Tan 1 X 1 X2 1 2 Wrt Cos 1 2x2 1 Explain In Great Detail Mathematics Topperlearning Com G4410njj

Find The Solution Set For Tan 1 X Cot 1 X Cos 1 2 X Study Com

2

If Cos 1 X 2 Cos 1 Y 3 A Then Prove That 9x 2 12xy Cos A 4y 2 36 Sin 2 A Sarthaks Econnect Largest Online Education Community

Incoming Term: cos inverse 1-x^2/1+x^2 formula,